Nossos serviços estão apresentando instabilidade no momento. Algumas informações podem não estar disponíveis.
Model for converting geometric altitudes (given by GNSS) into physical altitudes (compatible with the SGB´s Vertical Datum)
Description
Digital model that provides the factors to convert altitudes given by GNSS receivers, resulting in altitudes compatible with the terrestrial gravity, more suitable to describe the flow of liquid masses, like those needed in large irrigation projects, hydroelectric generation, etc.
In technical terms, a model to convert altitudes numerically describes the separation between the ellipsoid of reference of the geometric altitudes - also called ellipsoidal, resulting from the use of Global Navigation Satellite Systems (GNSS) - and the surface of reference of the physical altitudes, which is the vertical datum of the High-Precision Altimetric Network (RAAP) of the Brazilian Geodetic System (SGB), in the case of Brazil. From the geodetic coordinates resulting from the suitable processing of the observations collected through a geodetic GNSS receiver, the factor to convert geometric altitudes into physical altitudes should be extracted from the model.
The first models to convert altitudes published by the IBGE where the geoid models of the MAPGEO series, produced in partnership with the University of São Paulo (USP). MAPGEO92, MAPGEO2004, MAPGEO2010 and MAPGEO2015. The last one was the basis to develop the most recent model, hgeoHNOR2020, which opens a new series of models characterized by a better adaptation to the altitudes present in RAAP.
About the publication - Geoid Undulation Model
What is the Geoid?
In 1828, C.F. Gauss introduced an improved model of the figure of the Earth, but the term ‘geoid’ was created in 1873 by J.F. Listing. The Geoid is the equipotential surface of the Earth’s gravitational field, which coincides with the mean undisturbed sea level. Every point of this surface is at right angles to the gravity vector. The Geoid surface can be imagined as extended through the continents. It has a slightly irregular undulating shape that accompanies the structure variations of the Earth’s mass distribution. This undulation is about ±30 m, the maximum level is ±100m, in relation to the reference ellipsoid.
Why are geoid undulation models necessary?
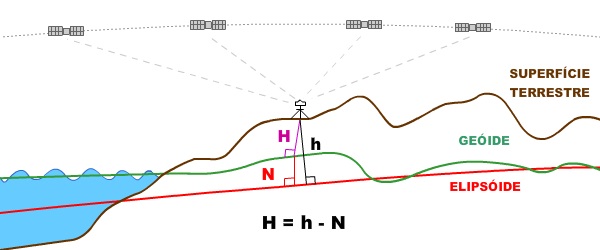
Heights determined by a GNSS receiver are not related to the mean sea level, but to a reference ellipsoid with specific dimensions. Therefore, it is necessary to know the geoid model (difference between geoid and ellipsoid surfaces) so that the height above mean sea level can be obtained.
Introduction
Due to its speed and precision obtaining coordinates, the Global Navigation Satellite Systems (GNSS) have revolutionized the activities which demand positioning. However, the height established using a GNSS receptor is not related to the mean sea level (or, more rigorously, to the geoid, which is the equipotential surface of the gravity field of the Earth that coincides with the mean still water level) but to an ellipsoidal reference with specific dimensions. As a result, it is necessary to tell the difference between the geoid surfaces and the ellipsoidal ones, i. e., the geoid height or undulation, in order to obtain the orthometric height (above the mean sea level). Thus, there is a great need for a more and more accurate Brazilian undulation model for applications in mapping and engineering. MAPGEO2015, as well as the previous models, (MAPGEO2010, MAPGEO2004, MAPGEO92), was designed to meet these demands. It was conceived in a partenership between the Brazilian Institute of Geography and Statistics (IBGE), through the Department of Geodetics (CGED), and the Polytechnic School of the University of São Paulo – EPUSP. The new model was estimated with a 5’-of-arc resolution, and the Geoid Undulation Interpolation System was updated. Via this system, users can obtain the geoid undulation at one point or a set of points, whose coordinates refer to SIRGAS2000 and are found between the latitudes 6°N and 35°S and between the longitudes 75°W and 30°W, inside the Brazilian territory.
In order to convert the ellipsoidal height (h), obtained by GNSS receptors, into orthometric height (H), it is necessary to use the value of the geoid height (N) obtained in a geoid undulation model, using the following expression:
More on the product - Geoid Undulation Model
MAPGEO2015
MAPGEO2015 is available for download either through a desktop Microsoft Windows application or online.
In both applications, the coordinates of the points for which the geoid undulation should be obtained can be entered either through a keyboard or file, according to the available formatting options of the entry and output.
Learn more - Geoid Undulation Model
Downloads
News and Releases
Model to Convert Altitudes
New IBGE application to convert altitudes improves precision and accelerates calculations
The IBGE launches today (5) an application with a new model to convert altitudes, the hgeoHNOR2020. Besides...
05/08/2021
Errata
Calendar error in release of Annual set of Planialtimetric Stations (GNSS) grounded on measured and processed RRNN
Published date: 29/07/2022
Description:
Error characterized for not meeting the release date of the product, according to the calendar available in December 2021. The product was canceled due to logistic and operational difficulties concerning the restriction of on-site activities in the first quarter for servants, most of them aged over 60 years, which led to restrictions in the production and further need to readjust deadlines. Therefore, the release of the Annual set of Planialtimetric Stations (GNSS) grounded on measured and processed RRNN was postponed from 2022 to 2023.Actions: The release calendar has been changed.
Processar os dados
!!ATENÇÃO!!
Em 5 de agosto de 2021, o IBGE lançará o novo modelo hgeoHNOR2020, que propicia maior acurácia na conversão entre altitudes geométricas (GNSS) e altitudes normais (RRNN do SGB). Em decorrência disso, a página de cálculo de alturas geoidais com o MAPGEO2015 (“MAPGEO2015 online”) não mais estará disponível.




